Let’s turn our attention to finding the domain of a function whose equation is provided. We will discuss interval notation in greater detail later. In interval notation, we use a square bracket. We can write the domain and range in interval notation, which uses values within brackets to describe a set of numbers. We can visualize the domain as a “holding area” that contains “raw materials” for a “function machine” and the range as another “holding area” for the machine’s products.
Or in a function expressed as a formula, we cannot include any input value in the domain that would lead us to divide by 0. For example, we cannot include any input value that leads us to take an even root of a negative number if the domain and range consist of real numbers. We also need to consider what is mathematically permitted. Keep in mind that, in determining domains and ranges, we need to consider what is physically possible or meaningful in real-world examples, such as tickets sales and year in the horror movie example above. In this section, we will practice determining domains and ranges for specific functions.
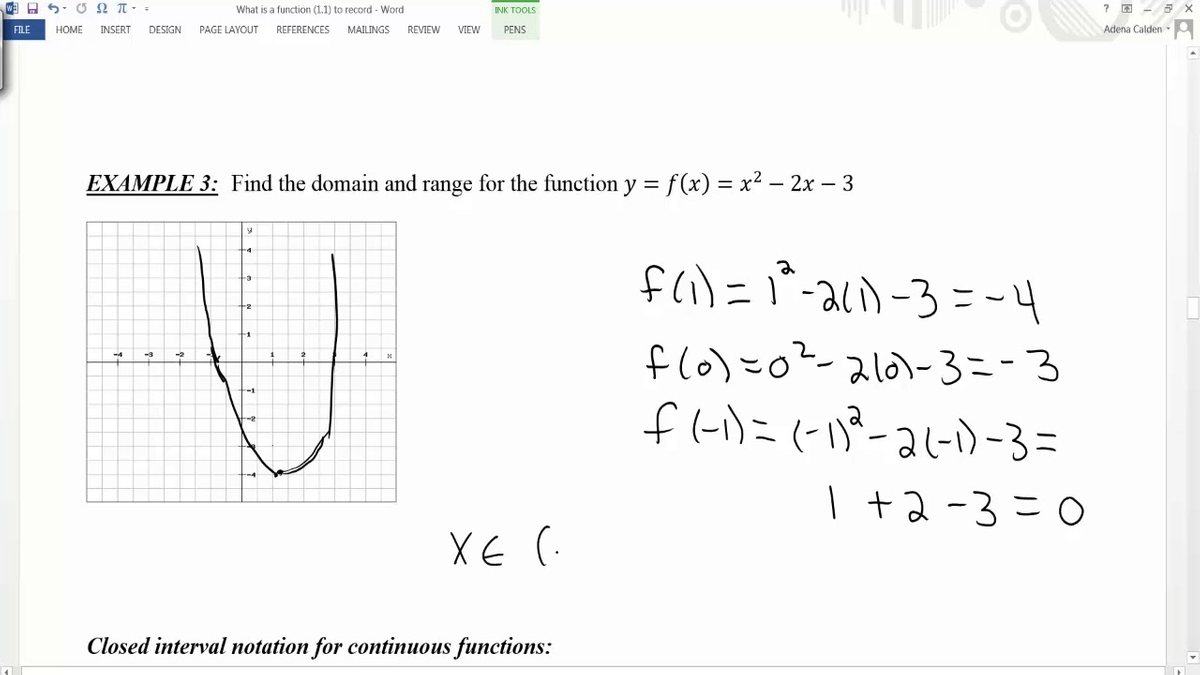
In Functions and Function Notation, we were introduced to the concepts of domain and range. Figure 1 Based on data compiled by 3 Finding the Domain of a Function Defined by an Equation